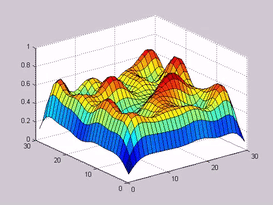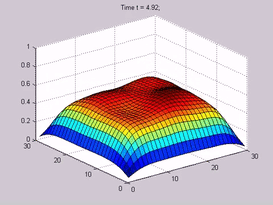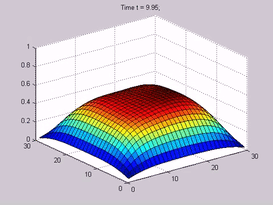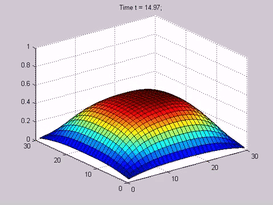
The heat equation is among the most widely studied topics in pure mathematics, and its analysis is regarded as fundamental to the broader field of partial differential equations. Solutions of the heat equation are sometimes known as caloric functions. The theory of the heat equation was first developed by Joseph Fourier in 1822 for the purpose of modeling how a quantity such as heat diffuses through a given region. The heat equation, along with variants thereof, is also important in many fields of science and applied mathematics. In probability theory, the heat equation is connected with the study of random walks and Brownian motion via the Fokker–Planck equation. The infamous Black–Scholes equation of financieal mathematics is a small variant of the heat equation, and the Schrödinger equation of quantum mechanics can be regarded as a heat equation in imaginary time.
For the better mathematical and physical understanding of the Heat Equation we should start by studying diffusion of heat.
The Diffusion Equation
We shall consider the typical parabolic equation Ut = k Uxx and its generalizations to two and three dimensions. The generalized one-dimensional diffusion equation k∇2U = Ut.
The Occurence of Diffusion Equation in Physics
The Conduction of Heat in Solids. If we denote by U the temperature at a point in a homogeneous isotropic solid, then it is readily shown that the rate of flow of heat per unit area across any plane is q = -kUn where k is thermal conductivity of the solid. Considering the flow of heat through a small element of volume, we can show that the variation of U is governed by the equation ρcUt = div(k grad U) + H(r,U,t) where p is the density and c the specific heat of the solid, and H(r,U,t) dT is the amount of heat generated per unit time in the element dT situated at the point with position vector r.
The Heat Equation For A Finite Rod
We will consider the heat equation Ut = k Uxx where k is the heat constant. Now we take the initial conditions and boundary conditions for this situation.
| Initial Conditions | Boundary Conditions |
| U(x,0) = f(x) | U(0,t) = 0 = U(l,t) |
U is a function of time and distance.
U = X(x).T(t)
Ut = XT’
Uxx = X”T
XT’ = kX”T = λ
X” – λX = 0 and T’ – kλT = 0
There shall be three cases –
1. λ = 0
2. λ = α2
3. λ = –α2
Now we shall delve into a detailed discussion of each case. Starting with the boundary conditions.
U(0,t) = 0, from this we can imply,
X(0).T(t) = 0
X(0) = 0
U(l,t) = 0, from this we can imply,
X(l).T(t) = 0
X(l) = 0
CASE 1- λ = 0
X” – λX = 0 and T’ – kλT = 0
So we can imply,
X” = 0 and T’ = 0
X = C1x + C2
X(0) = 0 , X(l) = 0
C1 = C2 = 0 , X = 0 , U = 0 is trivial solution.
CASE 2- λ = α2
X” – α2X = 0 , T’ – kα2T = 0
X = C1eαx + C2e–αx
X(0) = 0 , X(l) = 0
C1 = C2 = 0 , U = 0 is trivial solution.
CASE 3- λ = -α2
X” + α2X = 0 , T’ + kα2T = 0
X = A cos (αx) + B sin (αx)
When, X(0) = 0 , A = 0
When, X(l) = 0 ,
B sin (αl) = 0 , B is not equal to zero.
sin (αl) = 0,
αl = nπ,
α = nπ/l
STAY TUNED…